The Logic Behind Irrational Numbers: Exploring The Meaning Of This Mathematical Concept

image source: https://www.pinterest.com

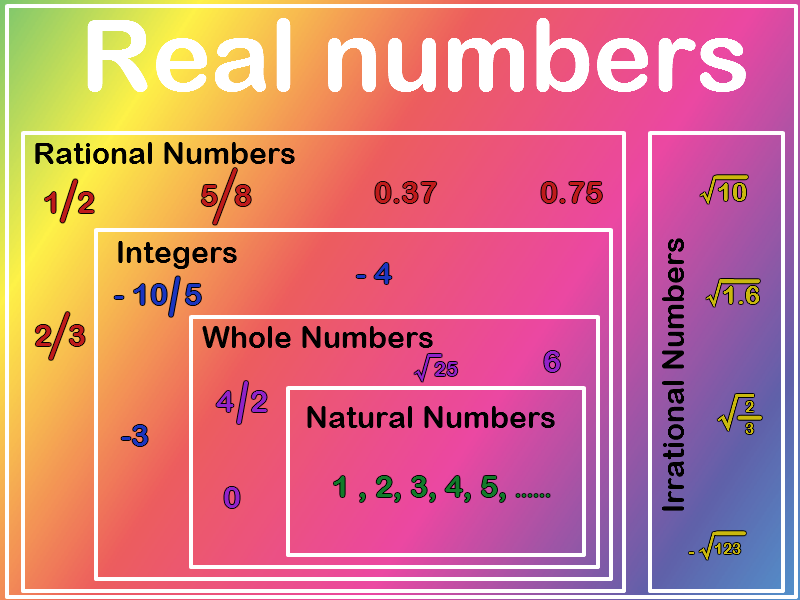
When it comes to numbers, there are two main categories: rational and irrational. While rational numbers can be expressed as a ratio of two integers, irrational numbers cannot. But what does it mean for a number to be irrational? In this article, we will explore the definition of irrational numbers and their significance in mathematics and everyday life. By the end, you will have a clear understanding of the concept and its relevance in both practical and theoretical contexts. So, let’s dive into the world of irrational numbers and discover their true meaning.

Unlocking the Mystery: Understanding What it Means for a Number to be Irrational

Irrational numbers are a fascinating and often misunderstood concept in mathematics. While most of us are familiar with whole numbers and decimals, irrational numbers can seem like a mysterious and complex topic. However, by delving into the meaning and properties of irrational numbers, we can unlock the mystery and gain a deeper understanding of this important mathematical concept.

First, let’s define what we mean by an irrational number. An irrational number is any number that cannot be expressed as a ratio of two integers (whole numbers). In other words, it is a number that cannot be written as a fraction or a terminating decimal. Some examples of irrational numbers include π (pi), √2 (the square root of 2), and √3 (the square root of 3).

One key property of irrational numbers is that they are infinite and non-repeating. This means that the decimal representation of an irrational number will continue on forever without repeating the same sequence of digits. For example, the decimal representation of π is 3.14159265358979323846264338327950288419716939937510…, with no pattern or repetition in the digits. This makes irrational numbers unique and intriguing, as they cannot be fully expressed or contained in our numerical system.

So why do irrational numbers exist? The answer lies in the relationship between numbers and geometry. In ancient Greece, mathematicians discovered that certain lengths, such as the diagonal of a square, could not be expressed as a ratio of two integers. These lengths were deemed “irrational” by the Greek mathematician Pythagoras. Today, we know that these lengths are irrational numbers, and they play a crucial role in geometry and other areas of mathematics.

In addition to their geometric significance, irrational numbers also have practical applications in the real world. For example, the number √2 is often used in measurements and construction to calculate the diagonal length of a square or the hypotenuse of a right triangle. The value of π is also essential for calculations in fields such as engineering, physics, and astronomy.

While irrational numbers may seem abstract and complex, they follow the same basic rules of arithmetic as rational numbers. They can be added, subtracted, multiplied, and divided, just like any other number. However, when irrational numbers are involved in a calculation, the result will always be another irrational number. For example, √2 + √3 = √5, which is still an irrational number.

In conclusion, irrational numbers are a fundamental and intriguing concept in mathematics. They are numbers that cannot be expressed as a fraction or a terminating decimal, and their decimal representation is infinite and non-repeating. Despite their mysterious nature, irrational numbers have real-world applications and play an essential role in geometry and other fields of mathematics. By understanding the properties and significance of irrational numbers, we can appreciate their beauty and complexity and use them to solve mathematical problems and explore the world around us.

Cracking the Code: Demystifying the Definition of Irrational Numbers

Irrational numbers have long been a source of confusion and mystery for many students of mathematics. These numbers, which cannot be expressed as a fraction of two integers, seem to defy our logical understanding of numbers. However, with a deeper understanding of their definition and properties, irrational numbers can be demystified and become more approachable.

Firstly, it is important to understand that irrational numbers are real numbers, just like rational numbers. Real numbers encompass all numbers on the number line, including positive and negative integers, fractions, decimals, and irrational numbers. The key difference between rational and irrational numbers lies in their representation. Rational numbers can be expressed as a ratio of two integers, while irrational numbers cannot.

One of the most well-known examples of an irrational number is pi (π). Pi is often approximated as 3.14, but in reality, its decimal representation is infinite and non-repeating. This means that it cannot be converted into a fraction of two integers, making it an irrational number. Other commonly known irrational numbers include the square root of 2 (√2), the golden ratio (φ), and Euler’s number (e).

So why do irrational numbers exist? The answer lies in the concept of irrationality itself. Rational numbers are a result of dividing one integer by another, and this process can continue indefinitely. However, not all infinite processes can be represented by rational numbers. For example, the decimal representation of √2 never repeats, but it also never ends. This means that it cannot be expressed as a fraction, making it an irrational number.

Now, it is worth noting that irrational numbers are not necessarily random or chaotic. In fact, they follow specific patterns and relationships, just like rational numbers. For instance, the decimal representation of π is an infinite non-repeating sequence, but it is also a transcendental number, meaning it cannot be the solution to any polynomial equation with rational coefficients. This shows that irrational numbers have their own unique properties and characteristics that distinguish them from rational numbers.

But why is it important to understand irrational numbers? For one, they play a significant role in calculations and measurements in fields such as science, engineering, and finance. For example, the value of π is crucial in calculations involving circles and spheres, while the golden ratio is often found in nature and used in architecture and design.

Furthermore, understanding irrational numbers can also deepen our understanding of mathematics as a whole. It challenges our thinking and encourages us to explore new concepts and ideas. It also highlights the beauty and complexity of mathematics, showing us that there is always more to discover and learn.

In conclusion, irrational numbers may seem elusive and perplexing at first, but with a clear definition and understanding of their properties, they can be demystified. These numbers may not fit into our traditional understanding of numbers, but they play an essential role in various fields and contribute to the richness and complexity of mathematics. So let us continue to embrace and explore these numbers, and crack the code of irrationality.

The Logic Behind Irrational Numbers: Exploring the Meaning of this Mathematical Concept

Irrational numbers are a fundamental concept in mathematics, yet their name can seem contradictory. How can a number be both irrational and still be considered a part of the mathematical world? In order to understand the logic behind irrational numbers, we must first define what they are.

An irrational number is any real number that cannot be expressed as a ratio of two integers. In other words, it cannot be written as a fraction where the numerator and denominator are both whole numbers. Some common examples of irrational numbers include pi (π), the square root of 2 (√2), and the golden ratio (φ). These numbers are infinite and non-repeating, making them unique and complex.

The concept of irrational numbers dates back to ancient Greece, with the discovery of the square root of 2 by Pythagoras. This discovery caused a great deal of controversy, as it challenged the belief held by many at the time that all numbers could be expressed as a ratio of integers. The name “irrational” was given to these numbers as a way to differentiate them from rational numbers, which can be written as fractions.

But why do we need to have a separate category for these numbers? Why not just consider them as another type of real number? The answer lies in the nature of irrational numbers themselves. Unlike rational numbers, which can be represented on a number line as a finite or repeating decimal, irrational numbers have an infinite and non-repeating decimal representation. This means that they cannot be expressed as a precise value, but rather as an approximation.

This unique property of irrational numbers has significant implications in mathematics. It means that we can never fully know or understand irrational numbers, as they are infinite and constantly changing. This challenges our understanding of the world and our ability to measure and quantify it. It also has practical applications, such as in the field of computer science where irrational numbers are used in algorithms to generate random numbers.

Furthermore, the existence of irrational numbers shows that mathematics is not just a human construct, but a fundamental aspect of the universe. The fact that irrational numbers can be found in nature, such as in the dimensions of a snowflake or the spiral of a seashell, suggests that they are a part of the natural order of things.

In conclusion, the logic behind irrational numbers lies in their infinite and non-repeating nature. They challenge our understanding of the world and have practical applications in various fields. Their existence also highlights the fundamental role of mathematics in the universe. So while the name “irrational” may seem contradictory, the concept of irrational numbers is a crucial part of the mathematical world.In conclusion, understanding what it means for a number to be irrational is crucial in mathematics and has significant implications in real-world applications. From its definition as a non-repeating and non-terminating decimal to its role in proving the existence of transcendental numbers, the concept of irrationality helps us comprehend the vastness and complexity of numbers. By recognizing and utilizing irrational numbers, we can unlock new possibilities and deepen our understanding of the world around us. As such, it is essential to grasp the meaning of irrationality and its significance in both theoretical and practical contexts.

Reference